Versão do R e dos pacotes minpack.lm e ggplot2.
R.version.string
#[1] "R version 4.0.1 (2020-06-06)"
packageVersion('minpack.lm')
#[1] ‘1.2.1’
packageVersion('ggplot2')
#[1] ‘3.3.0’
Versão do Ubuntu.
rui@rui:~$ lsb_release -a
No LSB modules are available.
Distributor ID: Ubuntu
Description: Ubuntu 20.04 LTS
Release: 20.04
Codename: focal
rui@rui:~$
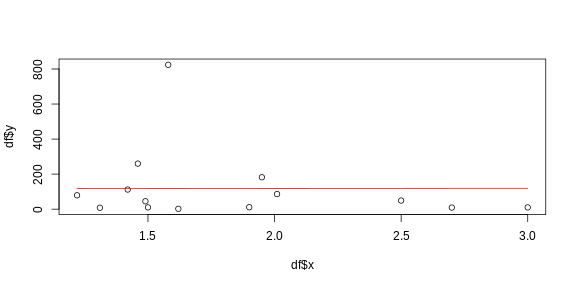
Ao tentar o código da resposta do
Carlos Eduardo Lagosta não obtive os mesmos resultados, o código deu um aviso de não convergência em 50 iterações.
Warning message:
In nls.lm(par = start, fn = FCT, jac = jac, control = control, lower = lower, :
lmdif: info = -1. Number of iterations has reached `maxiter' == 50.
E, portanto, os valores dos parâmetros ajustados foram diferentes.
fit
#Nonlinear regression model
# model: y ~ a * (1 - exp(-b * x))^c
# data: df
# a b c
# 6.783e+01 1.341e-06 -4.380e-02
# residual sum-of-squares: 605975
#
#Number of iterations till stop: 50
#Achieved convergence tolerance: 1.49e-08
#Reason stopped: Number of iterations has reached `maxiter' == 50.
Tentei então com valores diferentes para maxiter e ptol, que podem ser passados a nlsLM através de control = nls.lm.control(.).
library(minpack.lm)
library(ggplot2)
eps <- .Machine$double.eps^0.5
fit <- nlsLM(y ~ a*(1 - exp(-b*x))^c, data = df,
start = list(a = max(df$y), b = 0.05, c = 1),
control = nls.lm.control(maxiter = 100, ptol = eps))
summary(fit)
#
#Formula: y ~ a * (1 - exp(-b * x))^c
#
#Parameters:
# Estimate Std. Error t value Pr(>|t|)
#a 1.297e-02 8.481e+01 0.000 1.000
#b 2.372e-05 1.715e-01 0.000 1.000
#c -9.049e-01 2.271e+00 -0.399 0.698
#
#Residual standard error: 231.9 on 11 degrees of freedom
#
#Number of iterations to convergence: 93
#Achieved convergence tolerance: 1.49e-08
#
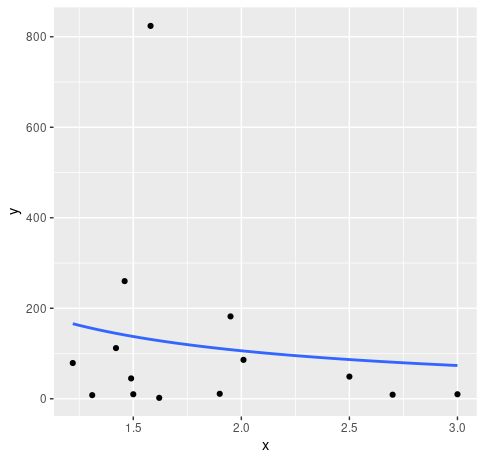
Agora o gráfico mas com o pacote ggplot seguindo o exemplo da resposta a esta pergunta.
ggplot(df, aes(x = x, y = y)) +
geom_point() +
geom_smooth(method = 'nlsLM',
formula = y ~ a*(1 - exp(-b*x))^c,
se = FALSE,
method.args = list(start = list(a = max(df$y), b = 0.05, c = 1),
algorithm = 'port',
control = nls.lm.control(maxiter = 100, ptol = eps)))